Flat-Top Beams and Plateau Uniformity Calculations
Beam Profiling Concepts | February 26, 2016
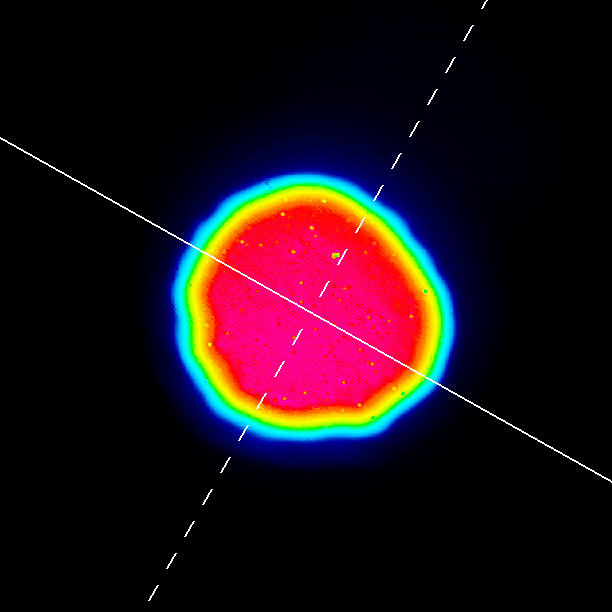
A flat-top beam (otherwise known as a top-hat) is used in various applications where a controlled profile is desired (i.e. a uniform density across the irradiated surface). Passing a Gaussian beam through special optics will create the flat-top beam and is the most common way of generating a flat-top beam. Uniform excimer beams are used in many material processing applications (e.g. creating integrated circuits). Industries such as welding, drilling, and medicine also make extensive use of flat-top beams. Although a rough estimate of a flat-top beam’s uniformity can be gauged visually using the image of the beam profile taken with a CCD or CMOS camera, a more accurate method is needed. The DataRay software (versions LCMvD23 and later) reports the plateau uniformity value, which gives the full width half maximum (FWHM) width of the beam’s power density histogram. The plateau uniformity of the flat-top beam is a value between zero and one used to quantify the uniformity of the flat-top beam. Zero represents a perfect flat-top beam. As the flat-top beam deviates from the theoretical flat-top, the value moves towards one. DataRay adheres to the ISO 13694 procedure for calculating the plateau uniformity. The plateau uniformity has the advantage of providing a single metric to characterize the beam uniformity across the entirety of the beam profile, rather than looking at individual slices of the beam.
Histogram
Mathematically a histogram (mi) is a function detailing the number of observations that can be placed into a bin of a certain width . Therefore, for a total number of observations n
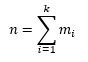
Where k is the number of bins. The histogram can be graphically represented by bars. The x-coordinates of each bar are defined by the bins position and width Δx while the y coordinates are defined by the value mi. In our application, the histogram takes the various pixel intensity values across the sensor and puts them into bins representing their intensity.
The number of observations is equal to the number of pixels and henceforth we will discard with the observation notation and refer only to pixels. The x-axis is the range of intensities across the sensor, and the height of the bars is determined by the number of pixels within the bars respective intensity range.
Plateau Uniformity
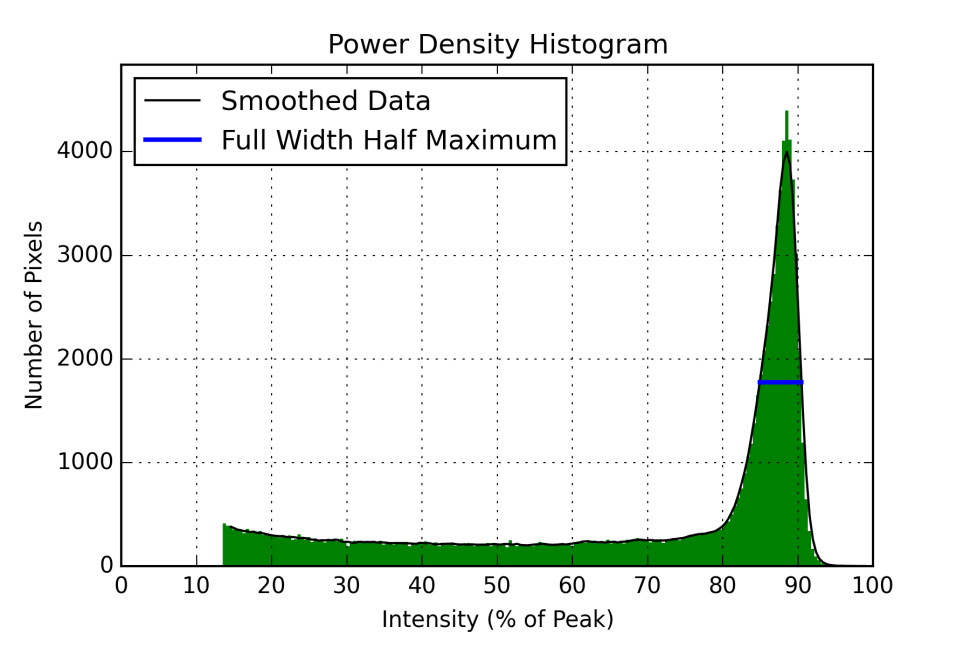
To determine the uniformity of the beam we take the FWHM value for the Gaussian distribution found on the histogram (see Figure 3). The ISO 13694 standard gives the general equation for determining the plateau uniformity for CW lasers as
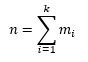
Where E(x,y,z) is the power density distribution. For pulse lasers, the equation is
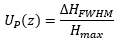
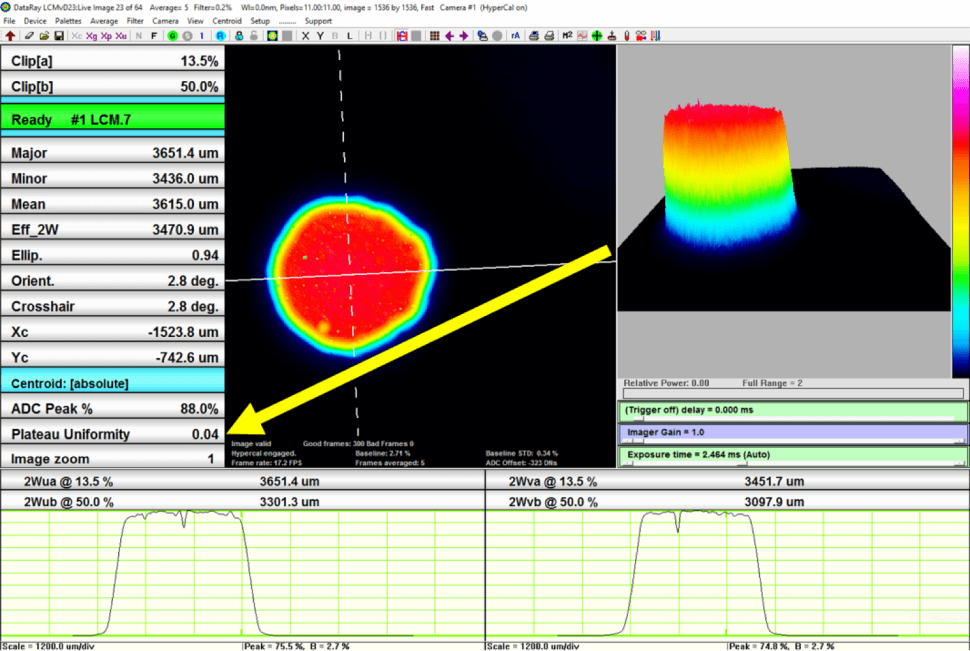
With the power density distribution replaced by the energy density distribution H(x,y,z). ΔEFWHM (ΔHFWHM) is the FWHM value near the peak Emax (Hmax) on the histogram. As the beam grows closer to the theoretical flat-top, Up (z) → 0. Since the plateau uniformity is a normalized value it follows that 0 < Up (z) < 1. The plateau uniformity measurement is not natively enabled in the DataRay software. To enable the measurement, click Setup in the menu bar. From the Setup menu, click the Enable Plateau Uniformity option. Once enabled, the plateau uniformity value can be found between the ADC Peak % button and the Image Zoom button (see Figure 2).
Cutoff
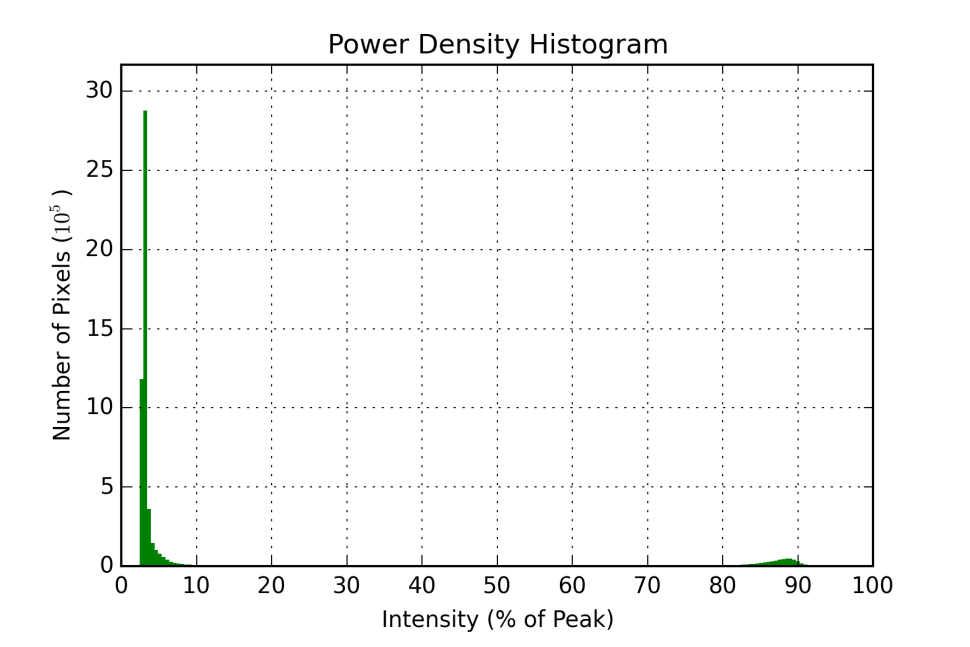
From the histogram of the flat-top beam, we see a large spike that dominates the histogram of the flat-top near 3% of the peak intensity (see Figure 3). This spike derives predominately from the unilluminated pixels on the sensor surface (see Figure 1). To remove the spike and look at meaningful data, only pixels whose values are greater than 13.5% of the peak intensity are used in the histogram (see Figure 3).
Theoretical Flat-Top Histograms
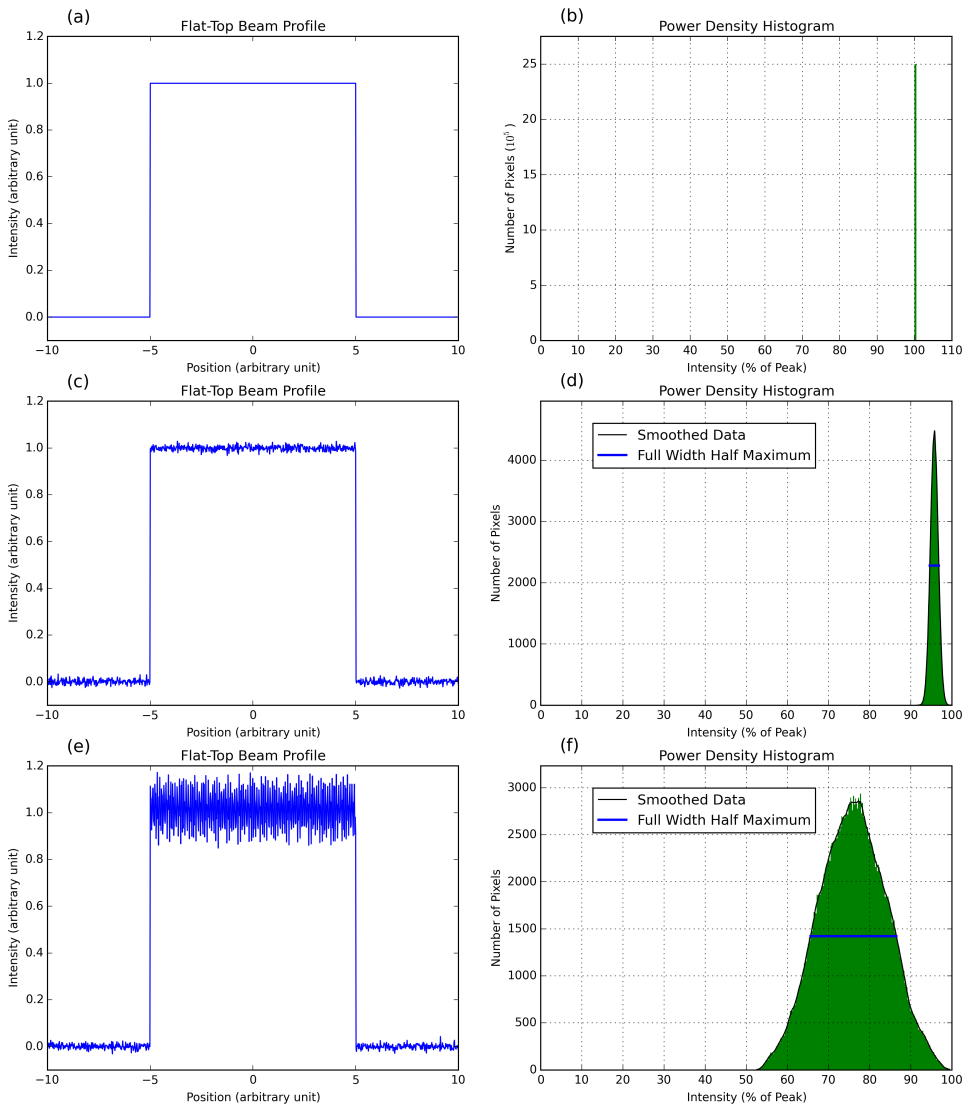
For a perfect flat-top beam (see Figure 5a), all the pixels have the same intensity value and are placed in a single bin (see Figure 5b). In this case, the plateau uniformity value is the width of the bin. Since Δx → 0. In reality, flat-top beams are almost never perfect. We generated a flat-top beam with Gaussian noise and its accompanying histogram (see Figure 5c and Figure 5d). The histogram now displays a Gaussian profile rather than the impulse spike. However, noise in the histogram profile can cause problems for determining the FWHM. DataRay software provides a smoothing algorithm (solid black) before looking for the FWHM. After smoothing the histogram data, the FWHM (solid blue) is easily calculated. For the flat-top with Gaussian noise, Up (z) → .022. However, a perfect flat-top beam with Gaussian noise is almost impossible to create. In practice a flat-top beam has different modes in the plateau region. We generated a flat-top beam with several different sinusoidal modes running along both the x and y axes (see Figure 5e). The Gaussian distribution begins to spread in the power density histogram (see Figure 5f) and we see that the Up (z) → .275. This series of generated flat-tops demonstrates the usefulness of the plateau uniformity measurement. The perfect flat-top has Up (z) = 0, the flat-top with Gaussian noise has Up (z) = 0.022, and the flat-top with sinusoidal modes has Up (z) = 0.275. Therefore, as the flat-top deviates from the perfect flat-top, Up (z) → 1.
Although the flat-tops generated show a perfect step when moving to and from a plateau region, with real optics a Gaussian like drop off in intensity is seen at the edges of the plateau (see Figure 1). Therefore, the histogram for a flat-top in real world conditions displays not only a Gaussian distribution, but lower level intensity values that come from the Gaussian component of the beam (see Figure 4). As long as the low level intensity values reside below the half maximum value, they will not affect the FWHM measurement calculation.
Application Note
An Application Note related to the topic described about is available for review here.
Should you have any further questions about DataRay’s plateau uniformity measurements feel free to contact us. Additionally, our product selector tool can help you find a laser beam profiling device well suited to your application, including cameras capable of plateau uniformity measurements. DataRay added this measurement to the software at the request of a customer. Please contact us if you would like any additional measurements added to the software. We are happy to work with our customers in order to improve our capabilities.
Author: Lucas Hofer
Related News
When to Use the D86 Beam Width Measurement Method
Beam Profiling Concepts |September 15, 2016
Although the clip level method and the second moment method are the most popular methods of beam width measurement, other beam width measurement techniques such as the D86 method can be used with beam profiling cameras.
Small Beam Width Theoretical and Experimental Error
Beam Profiling Concepts |March 14, 2016
One of the most important measurements in laser beam profiling is the beam width measurement. A common question we see is “How small of a beam can I measure with this camera?”
Systematic Error in ISO 11146 Measurements
Beam Profiling Concepts |May 12, 2016
Systematic error is an important calculation in any measurement system, and especially in ISO 11146 laser beam profiling measurements. In this blog post, we address the systematic error inherent to the measurement of beam width as laid out in the ISO 11146 standard.
